16 September, 2022

FX Options Guide - Section 19 - Barrier Option Intuition

Barrier Option Intuition Introduction

In this section we look to build on what we have learned in the previous barrier option sections by seeking to gain some barrier option intuition. To get the most from this section you should first have covered the sections on reverse knockout options, reverse knockin options, binary options and double knockout options, knockout options and knockout option risk management considerations.

Barrier Option Intuition

Comparing Barrier Options To Standard Options

The value of a standard option is dependent on the probability of spot finishing beyond the strike at expiration. The probability underlying a barrier option is a conditional probability such that the probability of finishing beyond strike at expiration is conditional upon having either touched or not touched the barrier level at any time prior to expiration.

Knockout Intuition

The value of a knockout option depends on the conditional probability of finishing beyond strike having not touched the outstrike prior to expiration.

As spot moves closer to the barrier:

• Probability of finishing beyond strike decreases
• Probability of touching the barrier increases
• Conditional probability decreases
• Price of the knockout option reduces

As spot moves away from the barrier:

• Probability of finishing beyond strike increases
• Probability of touching the barrier approaches zero
• Conditional probability approaches the probability of finishing beyond strike
• Price of the knockout option approaches that of the standard option

Knockin Intuition

The value of a knockin option depends on the probability of finishing beyond strike having touched the instrike prior to expiration. Therefore, in order for the knockin option to be triggered and then finish In-The-Money, spot has to first go in the direction of the barrier, trigger the barrier and then trade in the opposite direction until it has moved beyond strike.

As spot moves closer to the barrier:

• Probability of finishing beyond strike decreases
• Probability of touching the barrier increases
• Conditional probability increases, but as expiration approaches, the probability of finishing beyond strike approaches zero
• Price of the knockin option increases

As spot moves away from the barrier:

• Probability of finishing beyond strike increases
• Probability of touching the barrier approaches zero
• Conditional probability approaches zero
• Price of the knockin option approaches zero

Knockouts Vs Knockins

Reverse Knockout Intuition

The value of a reverse knockout option depends on the probability of finishing beyond strike having not touched the outstrike prior to expiration.

As spot moves closer to the barrier:

• Probability of finishing beyond strike increases
• Probability of touching the barrier increases
• Conditional probability increases until the probability of touching the barrier outweighs the probability of finishing beyond strike
• Value of the reverse knockout option increases until the barrier “touching” probability outweighs the strike “finishing” probability, then the value of the reverse knockout decreases

Barrier Intuition And Boundary Conditions

In trying to understand the value of a barrier option it is often useful to refer back to the options boundary condition.

• For a regular knockout option the most obvious boundary condition is the equivalent standard option
• For a reverse knockout option the equivalent standard option is a boundary condition, but it is not the most appropriate. Other possibilities:
  • Spread
  • Butterfly
  • 1x3x2 Butterfly

Probability Of Touching The Barrier

Clearly, the price of a knockout option is dependent on the probability of touching the barrier. For a standard option, intuition comes from the relationship between delta and the probability of finishing beyond strike. Delta (N(d1) in the Black-Scholes formula) is the hedge ratio of the option but is close enough to the probability of finishing beyond strike for intuition purposes (should really be looking at the strike delta N(d2) in the Blck-Scholes formula).

• The probability of touching a particular level is often approximately double the probability of finishing beyond the same level.
• So, looking at the OTM delta for a particular level and doubling the number gives you a rough rule of thumb guide to the probability of touching a particular level.

Probability Vs Delta

Consider the following example: EUR/USD, Maturity 3m, Spot 1.1400

Quoting Knockouts

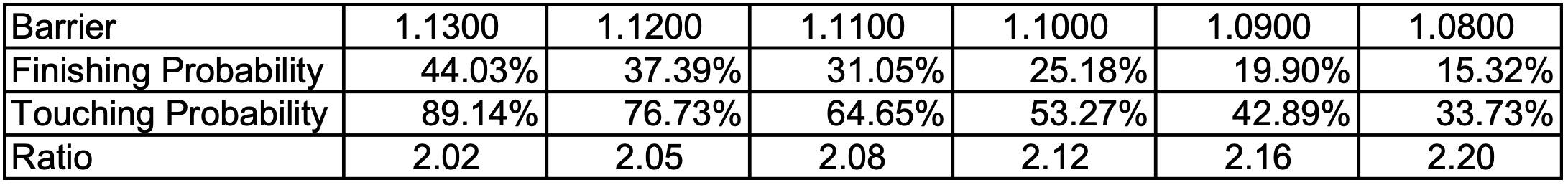
When asking for a price for a knockout option you need all the information you would normally need for a standard option and additionally you need to ask for the outstrike price. So you need to ask the client for:

• Expiration date
• Call currency
• Put currency
• Strike
• Outstrike
• Face amount

The knockout option can then be priced. The price will normally be quoted in terms of a percentage of one of the face amounts, or in terms of the amount of currency per one unit of the other currency. Unlike standard options, the price cannot be quoted in terms of volatility as unlike standard options there is no one pricing model that everybody uses. If the price was quoted in volatility terms the absolute currency amount of premiums would not always agree. Quotations are always in terms of the actual price.

The price that you receive will be based on the spot price entered into the pricing tool. The price will only be good whilst that spot price can be traded on, as the knockout option will need to be delta hedged. Therefore, the knockout option price can only be held out as long as the spot price can be held out. Normally, an indicative price on a particular spot reference will be given, and then if the client is interested, a firm price will be given based on a live spot price.

Once a trade is completed a confirmation will be faxed to the counterparty within twenty-four hours of the trade. The terms and conditions of the trade will be those of the price maker, in keeping with market convention.

Next Section - Barrier Option Risk Characteristics And Market Impact

In the next section we continue our introduction to barrier options by looking at barrier option risk characteristics and market impact.

---